Crave the Wave
| Crave the Wave | |||||||
|---|---|---|---|---|---|---|---|
| Type | Physics | ||||||
| Category | Study | ||||||
| Description | In this event competitors must demonstrate knowledge and process skills needed to solve problems and answer questions regarding all types of waves and wave motion. | ||||||
| Event Information | |||||||
| Latest Appearance | 2023 | ||||||
| Forum Threads | |||||||
| |||||||
| Question Marathon Threads | |||||||
| |||||||
| Division B Results | |||||||
| |||||||
In Crave the Wave, participants will demonstrate knowledge and process skills needed to solve problems and answer questions regarding all types and areas of waves and wave motion. A calculator is required for this event.
Regionals Topics
- General Wave Characteristics and Types
- Wave Phenomena
- Electromagnetic Waves Insert info on: energy carried (AM/FM only), standard wavelength bands, their uses and dangers, how the electromagnetic spectrum relates to everyday life, and mechanical and electromagnetic waves.
- Spectroscopy: Filters and Primary colors
Some calculations are required, such as calculation of frequency, period, wavelength, and speed.
General Wave Characteristics and Types
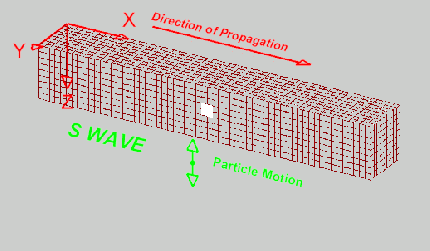
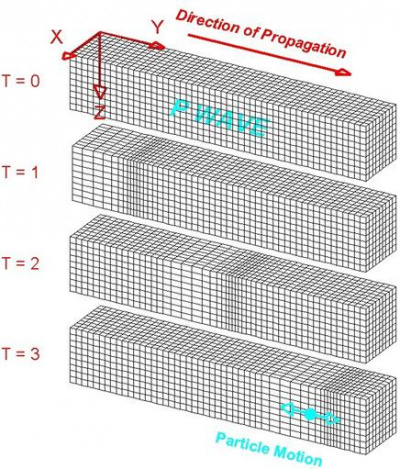
The two main types of waves are longitudinal (An example of this could be a P-wave) and transverse (An example of this could be an S-wave). Transverse waves propagate perpendicular to the direction of the wave. Longitudinal waves move parallel to the direction of the wave. These two wave types can be modeled with a Slinky. Start by having two people stretch a Slinky. To model a transverse wave, have one person shake their end of the Slinky with an up-and-down or a right-to-left motion. To model a longitudinal wave, keep the Slinky completely straight and have one person quickly push their end of the Slinky to the other person.
Other types of waves include surface waves and torsional waves. Surface waves are waves that travel along the boundary of two media. The particles in a surface wave move in a circular motion. Torsional waves twist and spin. It is like a screw being drilled or moving your arms back and forth while keeping them flat.
Transverse Waves
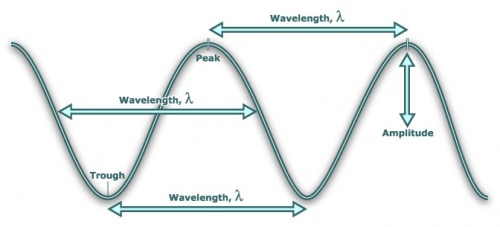
The main parts of a transverse wave are the crest, trough, wavelength, and amplitude. Also, there are additional characteristics of waves, such as frequency and period.
- Crest: the highest point of a wave. Also called peak.
- Trough: the lowest point of a wave.
- Rest position: the position the wave would be in if there were no disturbances along it. Also called normal position and equilibrium position.
- Wavelength: the distance between two crests or troughs. Measured by distance units of the metric system.
- Amplitude: the distance between a crest or trough and the rest position. Measured by distance units of the metric system.
- Frequency: the number of wavelengths passed per second. Measured in Hertz (Hz).
- Period: the time a wave takes to complete a wavelength. Measured in seconds.
- Direction of Motion: the direction in which the wave moves
- Direction of Oscillation: the direction in which particles in the wave move, perpendicular to the direction of motion
- Velocity: the speed and direction in which the wave is moving, equal to wavelength times frequency
Some of these parts are shown in the picture below:
Longitudinal Waves
- Compression: the most compressed point of a wave
- Rarefaction: the least compressed point of a wave
- Rest Position: the position the wave would be in if there were no disturbances along it. Also called normal position and equilibrium position.
- Wavelength: distance between two compressions or rarefactions. Measured by distance units of the metric system.
- Frequency: the number of wavelengths passed per second. Measured in Hertz (Hz).
- Period: the time a wave takes to complete a wavelength. Measured in seconds.
- Direction of Motion: the direction in which the wave moves
- Direction of Oscillation: the direction in which particles in the wave move, parallel to the direction of motion
- Velocity: the speed and direction in which the wave is moving, equal to wavelength times frequency
Surface Waves
- Crest: the highest point of a wave. Also called peak.
- Trough: the lowest point of a wave
- Rest Position: the position the wave would be in if there were no disturbances along it. Also called normal position, equilibrium position, and still-water position
- Wavelength: distance between two crests or troughs. Measured by distance units of the metric system.
- Frequency: the number of wavelengths passed per second. Measured in Hertz (Hz).
- Period: the time a wave takes to complete a wavelength. Measured in seconds.
- Direction of Motion: the direction in which the wave moves
- Direction of Oscillation: the direction in which particles in the wave move, circular motion
- Wave Height: the vertical distance between a crest and a trough
- Velocity: the speed and direction in which the wave is moving, equal to wavelength times frequency
Torsional Waves
- Direction of Motion: the direction in which the wave moves
- Velocity: the speed and direction in which the wave is moving
- Direction of Oscillation: the direction in which particles of the wave move
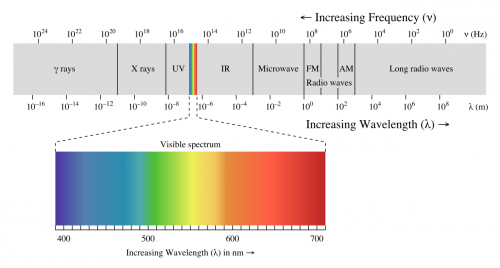
Electromagnetic Spectrum
The electromagnetic field is a combination of the magnetic field and the electric field. All EM waves are transverse in nature. They all travel at the speed of light, [math]\displaystyle{ c = 3.00 \times 10^8 \frac{m}{s} }[/math]. That means [math]\displaystyle{ \lambda \propto \frac{1}{f} }[/math], as [math]\displaystyle{ v = \lambda f }[/math] and [math]\displaystyle{ v = 3.00 \times 10^8 \frac{m}{s} }[/math]. The photon energy of a wave is measured in joules and electron volts and can be calculated as follows: [math]\displaystyle{ e = hf = \frac{ch}{\lambda} }[/math] where h = Planck's constant = [math]\displaystyle{ 6.62607 \times 10^{-34} Js }[/math]. Radio waves are the waves with the least energy, longest wavelength, and smallest frequency. These can be split up into AM waves, FM waves, short radio waves, telemetry/millimeter-waves, and terahertz waves. Next are microwaves which are used in microwaves to heat food and infrared waves which humans emit. After that are visible light waves which things like lava emit. Ultraviolet rays are next. Then come x-rays and gamma rays which have the highest energies.
Spectroscopy
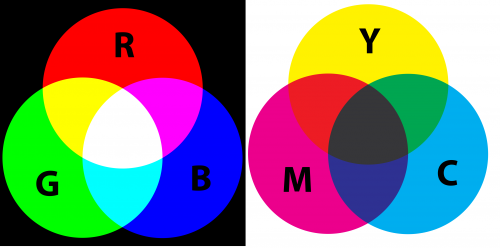
Colors
The primary colors of light are red, green, and blue, and the secondary colors of light are yellow, cyan, and magenta. Conversely, the primary colors of pigments are yellow, cyan, and magenta, and the secondary colors of pigments are red, green, and blue.
Filters
Filters: absorptive and dichroic/interference/thin film/reflective
Absorptive filters are usually made of glass with several compounds added which absorb specific wavelengths of light. They can also be made of plastic, which the compounds are added to, to produce gel filters.
Dichroic filters have little reflective cavities which resonate with specific wavelengths. Using destructive interference, the wavelengths are cancelled out, leaving the rest of the wavelengths to pass through. They are used for precise scientific work since their exact color range can be controlled. Interference filters are more expensive and more delicate.
Long-Pass Filter: Transmits waves with wavelengths longer than a specific range, Attenuates waves with shorter wavelengths
Short-Pass Filter: Transmits waves with wavelengths shorter than a specific range, Attenuates waves with longer wavelengths
Bandpass-Filter: Combination of long-pass and short-pass filters, transmits waves with a wavelength in a specific interval
Monochromatic Filter: Only a small range (usually one color) of wavelengths is allowed to pass.
Infrared Filter: The term can refer to infrared-passing or infrared cut-off filters, infrared photography (passing), projectors (cut-off),
Ultraviolet Filter: Block ultraviolet rays but transmit visible light rays, ultraviolet pass and ultraviolet bandpass filters are much less common, used in cameras
Neutral Density Filter: Attenuate all wavelengths of visible light, optical density is the common logarithm of the transmission coefficient, which is [math]\displaystyle{ amplitude_{initial}:amplitude_{incident} }[/math] or [math]\displaystyle{ intensity_{initial}:intensity_{incident} }[/math], make photographic exposures longer
Polarizer Filter: Blocks light depending on its polarization, usually made of Polaroid, sunglasses and photography, darker color
Spectra
Spectra are an application of the visible light spectrum to specific materials. Since certain materials have a unique absorption spectrum and emission spectrum associated with them, spectra can be used to identify unknown materials. They can also be used to learn more about materials at a microscopic level, including things such as molecular structure, crystal structure, and purity.
- Absorption spectra represent the portions of the spectrum that consist of wavelengths of incident radiation absorbed by the material. They are helpful in chemical analysis of stars (determining what they are made of and what quantity). Here is an example absorption spectrum.
- Emission spectra represent the portions of the spectrum that are emitted from a material when electrons from the atom are excited (e.g., from being heated). They are helpful in determining the composition of stars. Here is an example emission spectrum.
Absorption and emission spectra are very closely related, and there are theoretical models that exist where an absorption spectrum can be used to calculate a theoretical emission spectrum, and the other way around. However, this process is beyond the scope of Crave the Wave, so this task is unlikely to be on a test; however, tests may ask what these spectra can be used for, so it is important to know that this technique exists.
Wave Phenomena
Interference
Two waves strike each other.
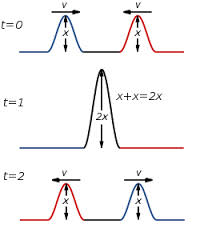
Constructive
The two waves reinforce each other.
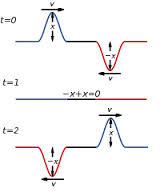
Destructive
The two waves cancel out each other.
Doppler Effect
The Doppler Effect is a difference in the frequency of a wave caused by a source moving relative to the observer. The Doppler Effect occurs because when an object emitting a wave is moving, the crests of the wave will bunch up in front of the object and the crests of the wave will be more spread out behind it. This causes a higher frequency of a wave when its source moves towards the observer and a lower frequency of a wave when its source moves away from the observer. An example of this is a siren passing by. It will sound higher pitched as it approaches, but then sound lower after it passes. The following equation is used to calculate the frequency of a wave using the Doppler effect:
[math]\displaystyle{ f }[/math] is the observed frequency, [math]\displaystyle{ f_0 }[/math] is the emitted frequency, [math]\displaystyle{ c }[/math] is the wave's velocity in the medium, [math]\displaystyle{ v_r }[/math] is the velocity of the observer relative to the medium, it is positive when the observer moves towards the source and vice versa, [math]\displaystyle{ v_s }[/math] is the velocity of the source relative to the medium, it is positive when the source moves away from the observer and vice versa.
Standing Waves
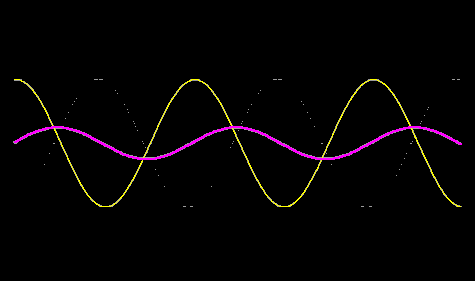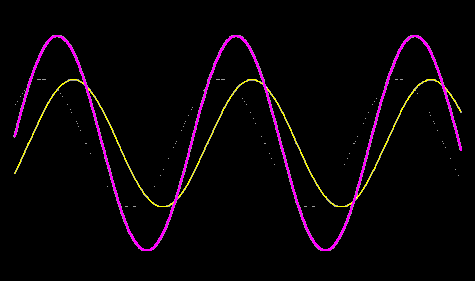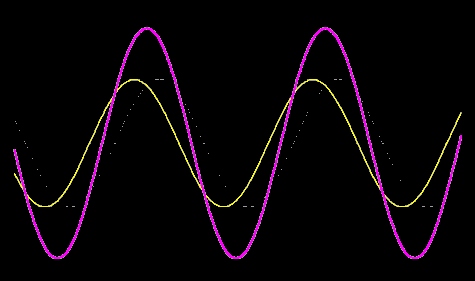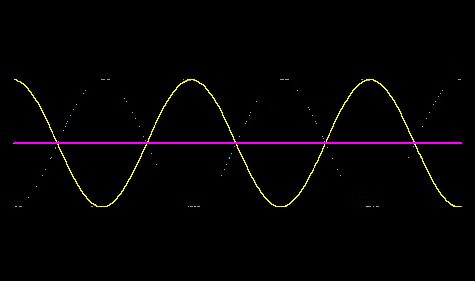
Standing waves occur when a wave does not appear to be made up of moving waves. This is from a combination of reflection and interference. Nodes are points of maximum destructive interference while anti-nodes are points of maximum constructive interference. The animation below shows the standing wave in pink, the initial wave in yellow, and the reflected beam in cyan (dotted):
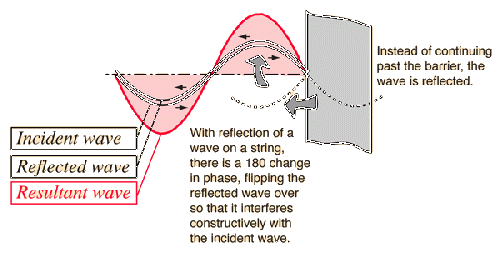
The following image shows how a standing wave can be simulated by attaching a string to a barrier, like a wall, and then moving it up and down. As the string is moved up and down, it forms a wave, but the wave stops at the barrier instead of going past it. When the wave stops, it is reflected back towards where the string is being moved, which causes a 180-degree shift in phase. This causes the interference in the wave necessary for a standing wave. This experiment can be tried at home with simple materials and by attaching the string to a rigid object, such as a hook or a doorknob of a closed door.
Reflection
The change of direction so that the wave bounces off into the same medium in which it originated.
Specular
If the reflection interface is very smooth, specular reflection will occur. Specular reflection is mirror-like (forms images).
Laws of reflection:
The normal, incident ray, and reflected ray all lie on the same plane.
The incident ray is the same angle from the normal as the reflected ray: [math]\displaystyle{ \theta_i = \theta_r }[/math]
The incident ray and reflected ray are on opposite sides on the normal.
An image in a glass mirror is 1) virtual, 2) reversed, 3) the right side up, 4) the same size of the object, and 5) the same distance behind the mirror as the object is in front of the mirror.
Diffuse
If the reflection interface is rough (non-metallic), diffuse reflection will occur. Diffuse reflection is where an incident ray is reflected at many different angles as opposed to specular reflection with only one angle of reflection. The visibility of objects is primarily due to diffuse reflection. Diffuse interreflection occurs when light reflected off a nearby object reflects off surrounding objects, illuminating them.
Refraction
Refraction is the change in direction of a wave caused by a change in its medium. Refraction is responsible for rainbows and mirages.
Snell's Law
The index of refraction, also called refractive index, is a measure of how light refracts in a medium. Its formula is:
n is the refractive index, c is the speed of light in a vacuum, and v is the speed of light in that medium.
Snell's Law states that the ratio of the velocities of a wave in two media is equal to the ratio of the sine of the angles of incidence, which is also equal to the reciprocal of the ratio of the refractive indices. The formula is:
[math]\displaystyle{ \theta }[/math] is the angle of incidence/reflection, which is measured from the normal, v is the velocity out of/in the medium, and n is the refractive index. Angles are measured from the normal.
Apparent Depth
When refraction occurs, velocity changes and frequency remains the same, so the wavelength of the wave must change because [math]\displaystyle{ v=\lambda f }[/math]. In general, the wave is partially reflected and partially refracted. The ratio of real depth to apparent depth is the ratio of the refractive index of water to that of air.
Kerr and Pockels Effects
The Kerr Effect is the change of refractive index of a material because of an applied electric field. [math]\displaystyle{ \Delta n \propto E^2 }[/math], meaning the change in the refractive index is proportional to the square of the energy of the electric field. The Kerr Electro-Optic Effect, or DC Kerr Effect, is when a slow varying electric field is applied. This makes the material birefringent, meaning the material shows different indices of radiation for light polarized parallel and perpendicular to the electric field. The difference is shown by the equation:
where [math]\displaystyle{ \Delta n }[/math] is the change in the index of reflection, [math]\displaystyle{ \lambda }[/math] is the wavelength of light, K is the Kerr Constant for the material, and E is the energy of the electric field.
The Optical Kerr Effect, or AC Kerr Effect, is when the electric field is due to the light itself. The Magneto-Optic Kerr Effect is when light reflected from a magnetized object has a slightly rotated plane of polarization.
The Pockels Effect is the change of refractive index of a crystal that does not show inversion symmetry, such as lithium niobate and gallium arsenide, because of an applied electric field. It differs from the Kerr effect in that [math]\displaystyle{ \Delta n \propto E }[/math], meaning the change in the refractive index is linear instead of quadratic.
Refractive Indices
Below is a large table of refractive indices of common materials.
| Material | Refractive Index |
|---|---|
| Vacuum | 1 |
| Gases at 0 °C and 1 atm | |
| Air | 1.000293 |
| Carbon Dioxide | 1.00045 |
| Helium | 1.000036 |
| Hydrogen | 1.000132 |
| Liquids at 20 °C | |
| Arsenic Trisulfide and Sulfur in Methylene Iodide | 1.9 |
| Benzene | 1.501 |
| Carbon Disulfide | 1.628 |
| Carbon Trichloride | 1.461 |
| Ethyl Alcohol (Ethanol) | 1.361 |
| Silicone Oil | 1.336-1.582 |
| Water | 1.3330 |
| 10% Glucose Solution in Water | 1.3477 |
| 20% Glucose Solution in Water | 1.3635 |
| 60% Glucose Solution in Water | 1.4394 |
| Solids at Room Temperature | |
| Titanium Dioxide (Rutile Phase) | 2.614 |
| Diamond | 2.419 |
| Strontium Titanate | 2.41 |
| Amber | 1.55 |
| Fused Silica (Fused Quartz) | 1.458 |
| Sodium Chloride | 1.544 |
| Other | |
| Liquid Helium | 1.025 |
| Water Ice | 1.31 |
| Cornea (human) | 1.373-1.401 |
| Lens (human) | 1.386-1.406 |
| Acetone | 1.36 |
| Ethanol | 1.36 |
| Glycerol | 1.4729 |
| Bromine | 1.661 |
| Teflon AF | 1.315 |
| Teflon | 1.35-1.38 |
| Cytop | 1.34 |
| Sylgard 184 (Polydimethylsiloxane) | 1.4118 |
| Polylactic acid | 1.46 |
| Acrylic glass | 1.490 - 1.492 |
| Polycarbonate | 1.584 - 1.586 |
| PMMA | 1.4893 - 1.4899 |
| PETg | 1.57 |
| PET | 1.5750 |
| Kerosene | 1.39 |
| Crown glass (pure) | 1.50 - 1.54 |
| Flint glass (pure) | 1.60 - 1.62 |
| Crown glass (impure) | 1.485 - 1.755 |
| Flint glass (impure) | 1.523 - 1.925 |
| Pyrex (a borosilicate glass) | 1.470 |
| Cryolite | 1.338 |
| Rock salt | 1.516 |
| Sapphire | 1.762–1.778 |
| Sugar Solution, 25% | 1.3723 |
| Sugar Solution, 50% | 1.4200 |
| Cubic zirconia | 2.15 - 2.18 |
| Potassium niobate ([math]\displaystyle{ KNbO_3 }[/math]) | 2.28 |
| Silicon carbide | 2.65 - 2.69 |
| Cinnabar (Mercury sulfide) | 3.02 |
| Gallium(III) phosphide | 3.5 |
| Gallium(III) arsenide | 3.927 |
| Zinc Oxide | 2.4 |
| Germanium | 4.05 - 4.01 |
| Silicon | 3.48 - 3.42 |
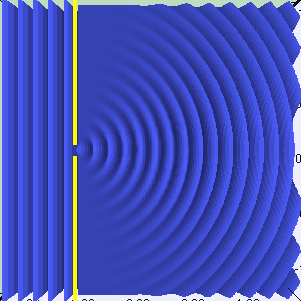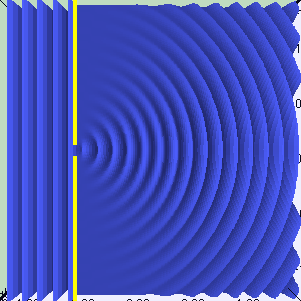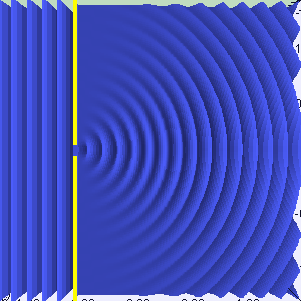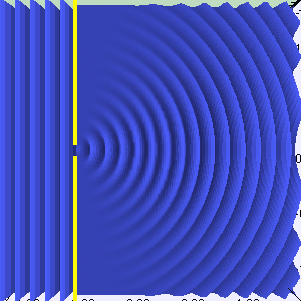
Diffraction
Diffraction is when a wave spreads out when encountering a corner or hole, such as a doorway or slit, that is comparable to its wavelength. Francesco Maria Grimaldi, an Italian scientist, coined the word 'diffraction.' Diffraction is described by the Huygens-Fresnel Principle.
State Topics
At States you will also need to demonstrate your knowledge about seismic waves.
Seismic Waves
There are 5 major seismic waves:
- P-waves: aka primary waves, are longitudinal waves. They are the first to arrive. They can travel through liquids.
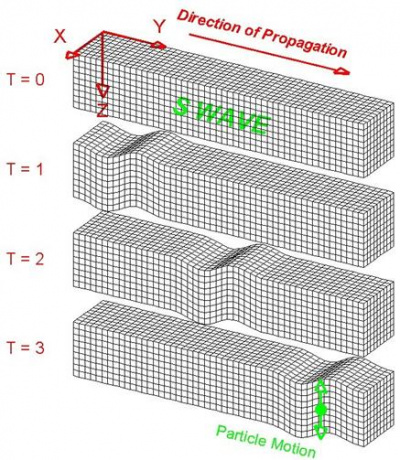
- S-waves: aka secondary or shear waves, are transverse waves. They are second to arrive. They cannot travel through liquids.
- Surface Waves: combinations of P and S waves and occur on the surface. They are the slowest waves.
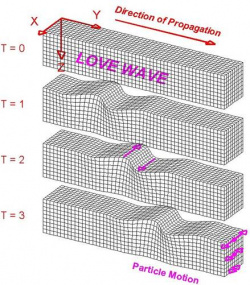
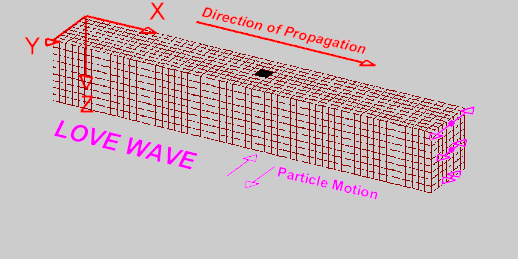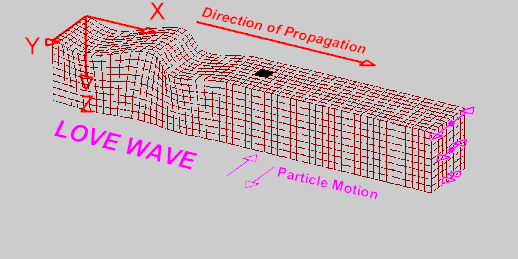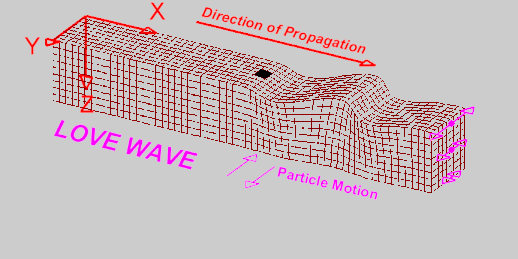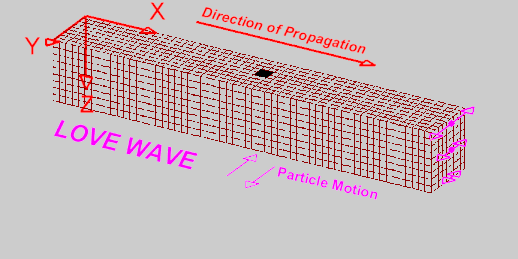
- Love Waves: waves that move in side to side, horizontally. Love waves cause the most damage.
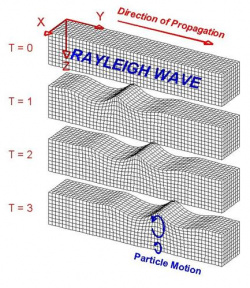
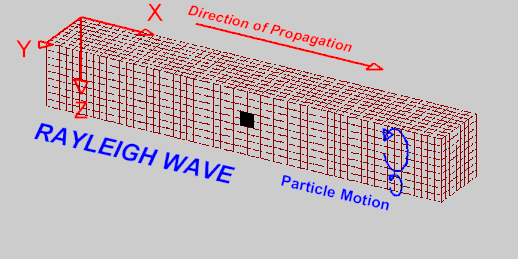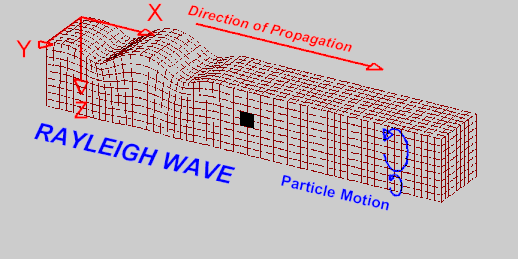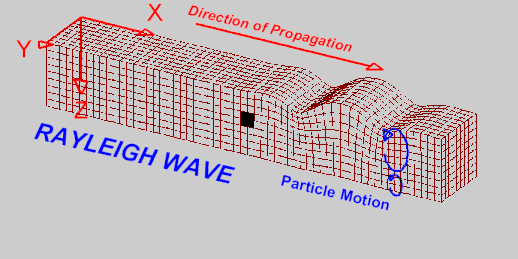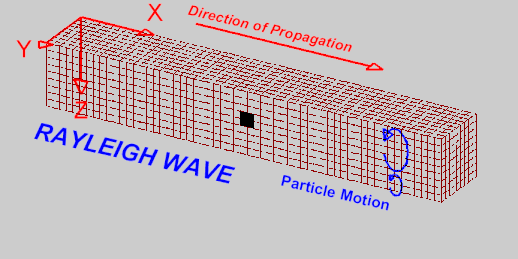
- Rayleigh Waves: waves that roll in an ocean-like motion.
National Topics
In addition to the Regional and State topics, Nationals will introduce boundary effects, such as breaking ocean waves and Tsunamis.
Breaking Ocean Waves
Breaking ocean waves occur when the amplitude of the wave reaches the point where the crest of the wave overturns.
Spilling Breakers
Spilling breakers are produced when the floor has a gradual slope. The crest becomes unstable and results in whitewater (foam), and the energy is released gradually. They take the longest to break and are some of the gentler waves. Onshore winds may cause more spilling breakers.
Plunging Breakers
Plunging waves are produced during a sudden depth change or a steep ocean floor. The crest turns over and crashes into the trough of the wave. Compressed air under the wave's lip creates the characteristic crashing sound. Most of its energy is released in one impact, and offshore winds may cause more plunging breakers.
Collapsing Breakers
Collapsing waves are a mix between plunging and surging breakers. The crest never fully breaks, but the bottom face of the wave collapses, producing whitewater.
Surging Breakers
Surging breakers occur at steep floors with long period waves. Because the base moves fast, the crest almost disappears. The wave has little to no breakage and not much whitewater is produced.
Tsunamis
A tsunami, or seismic sea wave, is a series of ocean waves that can be caused by things such as earthquakes or volcanic activity.
Notable Tsunamis
The earthquake and tsunami in the Indian Ocean on December 26, 2004, was the most devastating tsunami ever recorded. The estimated amount of casualties was 280,000 people.
Japan has a history of tsunamis, but a recent one was the earthquake of the coast of Japan on March 11, 2011. The tsunami is probably most known for the three nuclear reactors at the Fukushima Daiichi plant that had meltdowns, causing the meltdown to be declared the largest nuclear disaster since Chernobyl.
Equations to Know
There are several helpful formulas and equations to know.
Frequency and Period
- [math]\displaystyle{ f=\frac{1}{T} }[/math]
- f is the frequency, T is the period.
Rates
- [math]\displaystyle{ r=\frac{d}{t} }[/math]
- r is rate, d is distance, t is time.
Velocity
- [math]\displaystyle{ v=\lambda f }[/math]
- [math]\displaystyle{ v }[/math] is velocity, lambda ([math]\displaystyle{ \lambda }[/math]) is wavelength, [math]\displaystyle{ f }[/math] is frequency.
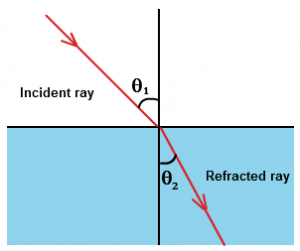
Snell's Law
- [math]\displaystyle{ \frac{\sin \theta_1}{\sin \theta_2}=\frac{v_1}{v_2} = \frac{n_2}{n_1} }[/math]
- It is helpful to use a picture for this equation:
- [math]\displaystyle{ \theta_1 }[/math] is the angle between the incident ray and the normal, [math]\displaystyle{ \theta_2 }[/math] is the angle between the refracted ray and the normal, [math]\displaystyle{ v_1 }[/math] is the velocity of light in the first medium (white), [math]\displaystyle{ v_2 }[/math] is the velocity of light in the second medium (blue), [math]\displaystyle{ n_1 }[/math] is the refractive index of the first medium (white), [math]\displaystyle{ n_2 }[/math] is the refractive index of the second medium (blue).
Rayleigh Criterion
- The Rayleigh Criterion estimates the angular resolution of an optical system, specifying the minimum separation between two light sources that can be resolved into distinct objects.
- Through a circular aperture, the equation is: [math]\displaystyle{ \theta=1.220\frac{\lambda}{D} }[/math]
- [math]\displaystyle{ \theta }[/math] is the angular resolution (in radians), [math]\displaystyle{ \lambda }[/math] is the wavelength of the light, and [math]\displaystyle{ D }[/math] is the diameter of the lens' aperture.
Young's Equation
- Young's Equation finds the wavelength of a light source relative to certain distances associated with a two-point light interference pattern.
- [math]\displaystyle{ \lambda=y*\frac{d}{m*L} }[/math]
- [math]\displaystyle{ \lambda }[/math] is the wavelength, [math]\displaystyle{ y }[/math] is the perpendicular distance from a point P on a nodal or antinodal line to a point on the central antinodal line, [math]\displaystyle{ d }[/math] is the distance between the slits or sources of light, [math]\displaystyle{ m }[/math] is the order value of the line P is on, [math]\displaystyle{ L }[/math] is the distance from point P to the sources of light.
Gratings
- A diffraction grating splits and diffracts light into many beams traveling in different directions and results in a characteristic rainbow-ish coloration. A grating typically has ridges on its surface. The grating equation relates the grating spacing and the angles of incident and diffracted light beams.
- [math]\displaystyle{ m\lambda=d(\sin \theta_i+\sin \theta_r) }[/math]
- [math]\displaystyle{ m }[/math] is the diffraction order, [math]\displaystyle{ \lambda }[/math] is the wavelength, [math]\displaystyle{ d }[/math] is the spacing of the grooves or slits, [math]\displaystyle{ \theta_i }[/math] is the angle of incidence, [math]\displaystyle{ \theta_2 }[/math] is the angle of diffraction.
Harmonics and Resonant Frequency
- [math]\displaystyle{ f_n = (n + 1) * f_0 }[/math]
- [math]\displaystyle{ f }[/math] is the nth harmonic frequency, [math]\displaystyle{ n }[/math] is the harmonic number, [math]\displaystyle{ f_0 }[/math] is the fundamental frequency.
Polarization - Malus' Law
- Malus' Law finds the intensity of light after passing through a linear polarizer.
- [math]\displaystyle{ I=I_0\cos^2 \theta_i }[/math]
- [math]\displaystyle{ I }[/math] is the intensity, [math]\displaystyle{ I_0 }[/math] is the initial intensity of the light, [math]\displaystyle{ \theta_i }[/math] is the angle between the light's initial polarization direction and the polarizer's axis.
Brewster's Angle
- Brewster's Angle is the angle of incidence where light with a certain polarization is transmitted through a transparent surface with no reflection.
- [math]\displaystyle{ \theta_i=\arctan \frac{n_2}{n_1} }[/math]
- [math]\displaystyle{ \theta_i }[/math] is the angle of incidence, [math]\displaystyle{ n_1 }[/math] is the first (incident) medium, [math]\displaystyle{ n_2 }[/math] is the second medium.
Energy of a Photon
- [math]\displaystyle{ E=hf=\frac{ch}{\lambda} }[/math]
- [math]\displaystyle{ E }[/math] is the energy of a photon, [math]\displaystyle{ h }[/math] is Planck's constant (approximately [math]\displaystyle{ 6.626*10^{-34} joule*s }[/math], c is the speed of light (approximately [math]\displaystyle{ 2.998*10^8 \frac{m}{s} }[/math], [math]\displaystyle{ \lambda }[/math] is the wavelength
- Alternatively, [math]\displaystyle{ E=\frac{1.24}{\lambda} }[/math], where [math]\displaystyle{ \lambda }[/math] is in micrometers and [math]\displaystyle{ E }[/math] comes out in electron-volts.
Dimensional Analysis
- Dimensional analysis is a method that uses base quantities to convert between various measurements. It is best explained through an example.
- Basic example: How many inches is 3 meters?
- First, convert from meters to centimeters. Set up the equation so that the meter measurements cancel out. [math]\displaystyle{ 3\,\text{meters} * \frac{100\,\text{centimeters}}{1\, \text{meter}} }[/math]
- Next, convert from centimeters to inches. Set up the equation so that the centimeter measurements cancel out. [math]\displaystyle{ 3\,meters*\frac{100\,centimeters}{1\,meter}*\frac{1\,inch}{2.54\,centimeters} }[/math]
- Notice that the meter and centimeter units cancel out. Thus, only the inch measurement is left. The answer then becomes [math]\displaystyle{ \frac{300}{2.54}\,inches }[/math], which equals [math]\displaystyle{ 118.11\,inches }[/math].
Sample Questions
- If the frequency of a light wave is 2 Hz (2 cycles per second), what is the wavelength?
- If the wavelength of a sound wave through air at 20 degrees Celsius is 2 cm, what is the frequency in Hz (cycles per second)?
- If the period is 2 seconds, what is the Frequency?
- What is Destructive Interference?
- Which seismic wave is faster? Primary waves or Secondary waves?
- Given an image of a wave, identify the wavelength.