Bottle Rocket B

[JasperKota]

Any idea of what times to expect from these rockets with the egg in them? They will be heavier than last years', so I don't know how to figure out of my design is any good.

My school just did tryouts, and to be honest everyone didn't do that great. I'm guessing 10 seconds will be considered to be OK, because no parachutes this time. The group who won (this is just tryouts for the team though) got an average of like 7, and they had a bottle on top of the pressurized one, and the top bottle had the egg inside it. On top of that they had a long clear tube, and then put an easter egg on top for the nose. It definitely helped with the backsliding, so you might consider that. Try throwing it up in the air really high, and if it nose dives, modify your design a little bit.

[Fanglin]

I found that a nose cone similarly to the one in the picture (above) is not very strong. What I did, is I took at fluorescent light cover, and rolled 0.07 plastic film around it to make a very lightweight tube, which you can then find a way to attach to the top of the bottle. Since this year requires an egg, I cut the first 6-10 inches of the top of another bottle, and attached that to the top of the other bottle. This adds a bit of weight, but creates a bunch of room to experiment with different kinds of padding etc.. To make the rocket as light as possible, I usually use a Mtn Dew/sierra mist bottle, packaging tape, and 0.07 film. (use stronger tape on the fins).

[Fanglin]

With this technique above, I can get about 20 seconds with a good rocket, with the egg having about a 75% survival rate.

[Fanglin]

What is the best length for the rocket and what's the best amount of water?

Most images in the image gallery show shorter rockets, but the pictures in Best of Nationals and elsewhere of high-performing rockets all seem to show longer rockets (not that I've ever taken this event, so my information probably has some errors).

The best length, is about 4-5 feet. I usually do about 30% water, although it does not really matter. (because of more water=less weight, and less water= less propulsion).

[andrewwski]

No - it actually does matter how much water you use!

Think about it this way - if you have all water and no air, you have no pressure. Nothing pushing the water out (except gravity, but then there's an equivalent volume of air entering upward at atmospheric pressure) - you won't go anywhere.

If you have all air and no water, you have a greater pressurized volume, but the only mass you are expelling is the mass of that air - you won't go very far.

There will actually be an optimum. You can think of two design variables to the optimization problem as being the pressurized volume and mass of propellant. You then have a constraint - a fixed volume - and thus you really have one degree of freedom - water-to-air ratio, assuming you're pumping to constant pressure.

If you look at the basic rocket equation, you have



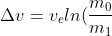where is the initial total mass (including propellant) and 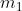is the dry mass (without propellant). So you could write



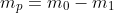
as the mass of the propellant, which allows (1) to be written as:



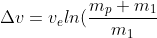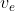is the escape velocity of the propellant, which will be dependent on the pressure. It's going to be time-varying, as the pressure will be time-varying, but a higher pressure will result in a higher .

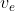We can take Bernoulli's equation, which says that the energy per unit volume must be conserved. For a single fluid:



where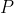 is pressure, 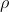is density of the fluid, and is velocity of the fluid. So, in our case, let's take state (1) to be the inside of the pressure vessel, where we have no velocity, and state (2) to be the edge of the nozzle, where pressure is ambient (gauge pressure is zero), and the fluid is moving out with escape velocity. So this reduces to:



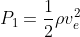
Now, of course, we lose pressure in the vessel as air is lost, so this is time varying, but you can see that it depends on the pressure.

Since the equation for the change in velocity - which is the quantity we want to optimize - is nonlinear, then we see that the ratio of water to air in the bottle does matter, as there is a ratio which will maximize .

We could further compute this theoretically by writing Bernoulli's equation for two fluids, writing a differential equation for the change in pressure over time, and then integrating over time, but it's probably easier to test it yourself by varying the amount of water.

[homesciencenerd]

Agreed, how much water you put in the bottle can change the end result.

[Fanglin]

No - it actually does matter how much water you use!

Think about it this way - if you have all water and no air, you have no pressure. Nothing pushing the water out (except gravity, but then there's an equivalent volume of air entering upward at atmospheric pressure) - you won't go anywhere.

If you have all air and no water, you have a greater pressurized volume, but the only mass you are expelling is the mass of that air - you won't go very far.

There will actually be an optimum. You can think of two design variables to the optimization problem as being the pressurized volume and mass of propellant. You then have a constraint - a fixed volume - and thus you really have one degree of freedom - water-to-air ratio, assuming you're pumping to constant pressure.

If you look at the basic rocket equation, you have



where is the initial total mass (including propellant) and is the dry mass (without propellant). So you could write



as the mass of the propellant, which allows (1) to be written as:



is the escape velocity of the propellant, which will be dependent on the pressure. It's going to be time-varying, as the pressure will be time-varying, but a higher pressure will result in a higher .

We can take Bernoulli's equation, which says that the energy per unit volume must be conserved. For a single fluid:



where is pressure, is density of the fluid, and is velocity of the fluid. So, in our case, let's take state (1) to be the inside of the pressure vessel, where we have no velocity, and state (2) to be the edge of the nozzle, where pressure is ambient (gauge pressure is zero), and the fluid is moving out with escape velocity. So this reduces to:



Now, of course, we lose pressure in the vessel as air is lost, so this is time varying, but you can see that it depends on the pressure.

Since the equation for the change in velocity - which is the quantity we want to optimize - is nonlinear, then we see that the ratio of water to air in the bottle does matter, as there is a ratio which will maximize .

We could further compute this theoretically by writing Bernoulli's equation for two fluids, writing a differential equation for the change in pressure over time, and then integrating over time, but it's probably easier to test it yourself by varying the amount of water.

Well obviously the extremes won't work, but I was talking about 10% to maybe 40%. I have tested a ton of the combinations, and there is not much deviation.

[homesciencenerd]

Not true, my partner and i had the best times with 37%. Compared to a whole 1 second difference when the bottle was filled with 30%, 15%, or 45%(ect,)

[deepali]

Where can i find the official rules for 2016.

[deepali]

Do we have to put egg at the nose of the bottle or it can be inside the bottle also.